Tích vô hướng của hai vectơ.

Tích vô hướng của hai vectơ

A. Lý thuyết

I. Định nghĩa

• Tích vô hướng hai vectơ \[\] và \[\] được tính theo công thức: .
• Chú ý: \[\overrightarrow{a}.\overrightarrow{a}={{\overrightarrow{a}}^{2}}={{\left| \overrightarrow{a} \right|}^{2}}\].

II. Biểu thức tọa độ của tích vô hướng

• Cho \[\overrightarrow{a}\left( {{a}_{1}},{{a}_{2}} \right),\overrightarrow{b}\left( {{b}_{1}},{{b}_{2}} \right)\]. Khi đó: \[\overrightarrow{a}.\overrightarrow{b}={{a}_{1}}.{{b}_{1}}+{{a}_{2}}.{{b}_{2}}\].
• \[\left| \overrightarrow{a} \right|=\sqrt{a_{1}^{2}+a_{2}^{2}}\]. Nếu \[\overrightarrow{a}\bot \overrightarrow{b}\] thì \[\overrightarrow{a}.\overrightarrow{b}=0\].
• \[\cos \left( \overrightarrow{a},\overrightarrow{b} \right)=\frac{\overrightarrow{a}.\overrightarrow{b}}{\sqrt{a_{1}^{2}+a_{2}^{2}}.\sqrt{b_{1}^{2}+b_{2}^{2}}}\].

B. Bài tập

I. Bài tập minh họa

Câu 1: Cho hai điểm A(4;3) và B(2;-1). Tìm điểm M trên trục hoành sao cho \[\left| \overrightarrow{MA}+\overrightarrow{MB} \right|\] đạt giá trị nhỏ nhất.             A. M(3;0)         B. M(2;0)         C. M(1;0)         D. M(0;0)

Lời giải: Chọn A.

\[M\in Ox\Leftrightarrow M\left( m,0 \right)\].

Nên \[\overrightarrow{MA}\left( m-4;3 \right);\overrightarrow{MB}\left( m-2;-1 \right)\Rightarrow \overrightarrow{MA}+\overrightarrow{MB}=(2m-6;2)\Rightarrow \left| \overrightarrow{MA}+\overrightarrow{MB} \right|=\sqrt{{{\left( 2m-6 \right)}^{2}}+4}\ge 2\].

Dấu bằng xảy ra khi m=3. Vậy M(3;0).

Câu 2: Cho \[\left| \overrightarrow{a} \right|=\left| \overrightarrow{b} \right|=1,\left| 2\overrightarrow{a}-\overrightarrow{b} \right|=\sqrt{3}\]. Tính \[\overrightarrow{a}.\overrightarrow{b}\].             A. 1                 B. \[\frac{1}{2}\]                     C.-1                 D. \[-\frac{1}{2}\]

Lời giải: Chọn B.

\[\left| 2\overrightarrow{a}-\overrightarrow{b} \right|=\sqrt{3}\Leftrightarrow {{\left| 2\overrightarrow{a}-\overrightarrow{b} \right|}^{2}}=3\Leftrightarrow 4{{\overrightarrow{a}}^{2}}+{{\overrightarrow{b}}^{2}}-4\overrightarrow{a}.\overrightarrow{b}=3\Leftrightarrow 4{{\left| \overrightarrow{a} \right|}^{2}}+{{\left| \overrightarrow{b} \right|}^{2}}-4\overrightarrow{a}.\overrightarrow{b}=3\]\[\Leftrightarrow 5-4\overrightarrow{a}.\overrightarrow{b}=3\Leftrightarrow \overrightarrow{a}.\overrightarrow{b}=\frac{1}{2}\].

Câu 3: Tính góc giữa hai vectơ thỏa mãn \[3\overrightarrow{a}-5\overrightarrow{b}\bot 2\overrightarrow{a}+\overrightarrow{b};\overrightarrow{a}+4\overrightarrow{b}\bot \overrightarrow{a}-\overrightarrow{b}\].             A. \[\frac{19}{3\sqrt{43}}\]    B. \[\frac{19}{2\sqrt{43}}\]    C. \[\frac{19}{5\sqrt{43}}\]         D.  \[\frac{19}{\sqrt{43}}\]

Lời giải: Chọn C.

\[3\overrightarrow{a}-5\overrightarrow{b}\bot 2\overrightarrow{a}+\overrightarrow{b}\Leftrightarrow \left( 3\overrightarrow{a}-5\overrightarrow{b} \right)\left( 2\overrightarrow{a}+\overrightarrow{b} \right)=0\Leftrightarrow 6{{\text{a}}^{2}}-5{{b}^{2}}-7\overrightarrow{a}.\overrightarrow{b}=0\Leftrightarrow \overrightarrow{a}.\overrightarrow{b}=\frac{6{{\text{a}}^{2}}-5{{b}^{2}}}{7}\left( 1 \right)\]

\[\overrightarrow{a}+4\overrightarrow{b}\bot \overrightarrow{a}-\overrightarrow{b}\Leftrightarrow \left( \overrightarrow{a}+4\overrightarrow{b} \right)\left( \overrightarrow{a}-\overrightarrow{b} \right)=0\Leftrightarrow {{a}^{2}}-4{{b}^{2}}+3\overrightarrow{a}.\overrightarrow{b}=0\Leftrightarrow \overrightarrow{a}.\overrightarrow{b}=\frac{4{{b}^{2}}-{{a}^{2}}}{3}\left( 2 \right)\]

\[\left( 1 \right)\left( 2 \right)\Rightarrow \frac{6{{\text{a}}^{2}}-5{{b}^{2}}}{7}=\frac{4{{b}^{2}}-{{a}^{2}}}{3}\Leftrightarrow {{b}^{2}}=\frac{25}{43}{{a}^{2}}\Rightarrow \overrightarrow{a}.\overrightarrow{b}=\frac{19}{43}{{a}^{2}}.\]

\[\cos \left( \overrightarrow{a},\overrightarrow{b} \right)=\frac{\overrightarrow{a}.\overrightarrow{b}}{\left| \overrightarrow{a} \right|.\left| \overrightarrow{b} \right|}=\frac{\overrightarrow{a}.\overrightarrow{b}}{\left| \overrightarrow{a} \right|.\left| \frac{5}{\sqrt{43}}\overrightarrow{a} \right|}=\frac{19}{43}{{a}^{2}}:\left( \frac{5}{\sqrt{43}}{{a}^{2}} \right)=\frac{19}{5\sqrt{43}}.\]

Câu 4: Cho tứ giác ABCD, m là điểm tùy ý. K là điểm cố định thỏa mãn đẳng thức \[\overrightarrow{MA}+\overrightarrow{MB}+\overrightarrow{MC}+3\overrightarrow{M\text{D}}=x\overrightarrow{MK}\]. Tìm x.             A. 2                 B. 5                  C. 6                  D. 8

Lời giải: Chọn C.

Gọi G là trọng tâm tam giác ABC và I là trung điểm DG thì ta có:

\[\overrightarrow{MA}+\overrightarrow{MB}+\overrightarrow{MC}+3\overrightarrow{M\text{D}}=3\overrightarrow{MG}+3\overrightarrow{M\text{D}}=3\left( \overrightarrow{MG}+\overrightarrow{M\text{D}} \right)=6\overrightarrow{MI}\]. Vậy \[6\overrightarrow{MI}=x\overrightarrow{MK}\]. Nên K chính là I và x=6.

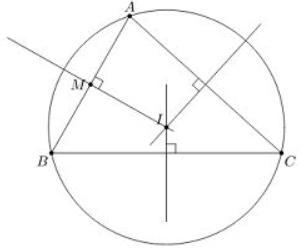
Câu 5: Cho hai điểm M, N nằm trên đường tròn đường kính AB=2R. Gọi I là giao điểm của hai đường thẳng AM và BN. Tính \[\overrightarrow{AM}.\overrightarrow{AI}+\overrightarrow{BN}.\overrightarrow{BI}\] theo R.             A. \[4{{R}^{2}}\]          B. \[3{{R}^{2}}\]                      C. \[2{{R}^{2}}\]                      D. \[{{R}^{2}}\]

Lời giải: Chọn A.

\[\overrightarrow{AM}.\overrightarrow{AI}=\left( \overrightarrow{AB}+\overrightarrow{BM} \right).\overrightarrow{AI}=\overrightarrow{AB}.\overrightarrow{AI}+\overrightarrow{BM}.\overrightarrow{AI}=\overrightarrow{AB}.\overrightarrow{AI}\]

\[\overrightarrow{BN}.\overrightarrow{BI}=\left( \overrightarrow{BA}+\overrightarrow{AN} \right).\overrightarrow{BI}=\overrightarrow{BA}.\overrightarrow{BI}+\overrightarrow{AN}.\overrightarrow{BI}=\overrightarrow{BA}.\overrightarrow{BI}\]

Nên \[\overrightarrow{AM}.\overrightarrow{AI}+\overrightarrow{BN}.\overrightarrow{BI}=\overrightarrow{AB}.\overrightarrow{AI}+\overrightarrow{BA}.\overrightarrow{BI}=\overrightarrow{AB}\left( \overrightarrow{AI}-\overrightarrow{BI} \right)=A{{B}^{2}}=4{{R}^{2}}\].

II. Bài tập tự luyện

Câu 1: Cho các vectơ \[\overrightarrow{a},\overrightarrow{b}\] thỏa mãn \[\left| \overrightarrow{a} \right|=8,\left| \overrightarrow{b} \right|=10,\left( \overrightarrow{a},\overrightarrow{b} \right)={{60}^{0}}\] .Tính \[\overrightarrow{a}.\overrightarrow{b}\].

            A. 40                B. 80                C. 12                D. 43

Câu 2: Chọn khẳng định đúng?

A. \[\exists \overrightarrow{a},{{\left| \overrightarrow{a} \right|}^{2}}={{\left| \overrightarrow{a} \right|}^{2}}\].

B. \[\forall \overrightarrow{a},{{\left| \overrightarrow{a} \right|}^{2}}={{\left| \overrightarrow{a} \right|}^{2}}\].

C. \[\forall \overrightarrow{a,}{{\left| \overrightarrow{a} \right|}^{2}}<0\].

D. \[\forall \overrightarrow{a,}{{\left| \overrightarrow{a} \right|}^{2}}>0\].

Câu 3: Cho tam giác ABC có AB=2, BC=4, CA=3. G là trọng tâm tam giác. Tính \[\overrightarrow{AG}.\overrightarrow{BC}\].

            A. \[\frac{5}{3}\]                    B. \[\frac{5}{4}\]                     C. \[\frac{5}{6}\]             D. \[\frac{5}{8}\]

Câu 4: Cho tam giác ABC có AB=2, BC=4, CA=3. G là trọng tâm tam giác. Tính \[\overrightarrow{GA}.\overrightarrow{GB}+\overrightarrow{GB}.\overrightarrow{GC}+\overrightarrow{GC}.\overrightarrow{GA}\].

            A. \[-\frac{29}{6}\]                 B. \[-\frac{29}{7}\]                 C. \[-\frac{28}{7}\]          D. \[-\frac{28}{51}\]

Câu 5: Cho hình vuông ABCD cạnh a. Tính \[\left( \overrightarrow{AB}+\overrightarrow{AC}+\overrightarrow{A\text{D}} \right)\left( \overrightarrow{DA}+\overrightarrow{DB}+\overrightarrow{DC} \right)\]

            A. 0                 B. 1                  C. 2                  D. 3

Câu 6: Cho tam giác ABC và M là một điểm bất kì. Tính \[\overrightarrow{MA}.\overrightarrow{BC}+\overrightarrow{MB}.\overrightarrow{CA}+\overrightarrow{MC}.\overrightarrow{AB}\].

            A. 0                 B. 1                  C. 2                  D. 3

Câu 7: Cho \[\left| \overrightarrow{a} \right|=5,\left| \overrightarrow{b} \right|=12,\left| \overrightarrow{a}+\overrightarrow{b} \right|=13\]. Tính \[\overrightarrow{a}\left( \overrightarrow{a}+\overrightarrow{b} \right)\].

            A. 14                B. 33                C. 25                D. 21

Câu 8: Cho nửa đường tròn tâm O đường kính AB=2R. Gọi M, N là hai điểm trên (O) và \[AM\cap BN=I\] .Tính \[\overrightarrow{AI}.\overrightarrow{AM}+\overrightarrow{BI}.\overrightarrow{BN}\].

             A. \[2{{\text{R}}^{2}}\]           B. \[\text{3}{{\text{R}}^{2}}\]             C. \[\text{4}{{\text{R}}^{2}}\]     D. \[\text{5}{{\text{R}}^{2}}\]

Câu 9: Cho tam giác ABC có ba đường trung tuyến AD, BE, CF. Tính \[\overrightarrow{BC}.\overrightarrow{A\text{D}}+\overrightarrow{CA}.\overrightarrow{BE}+\overrightarrow{AB}.\overrightarrow{CF}\].

            A. 0                 B. 1                  C. 2                  D. 3

Câu 10: Cho 4 điểm A, B, C, D tùy ý. Tính \[\overrightarrow{AB}.\overrightarrow{C\text{D}}+\overrightarrow{AC}.\overrightarrow{DB}+\overrightarrow{A\text{D}}.\overrightarrow{BC}\].

            A. 0                 B. 1                  C. 2                  D. 3

Đáp án bài tập tự luyện

 

Bài viết gợi ý: